Parallel links are one of the best and easiest ways to maintain level with the ground, but often we just need to keep the same angle. I once designed a holder for a seam welding robot that needed to keep the tip at a specific angle relative to the part. It was not horizontal.
The links in a parallel linkage system can be likened to the lines that form a parallelogram. Each corner is a pivot and throughout the motion, the lines will stay parallel. Proper design necessitates that the lengths of non-connecting members are equal.
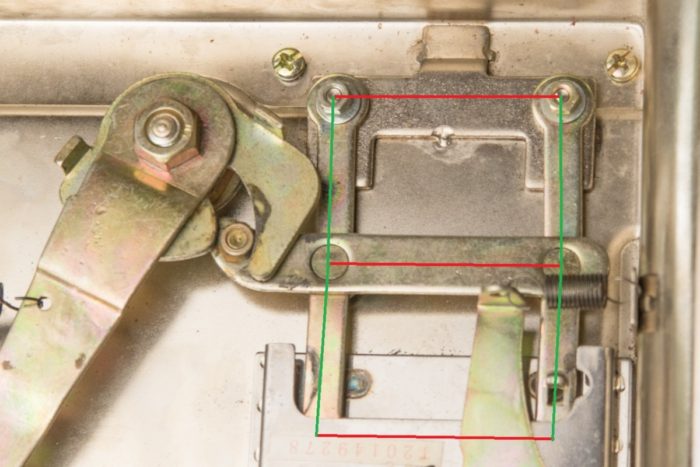
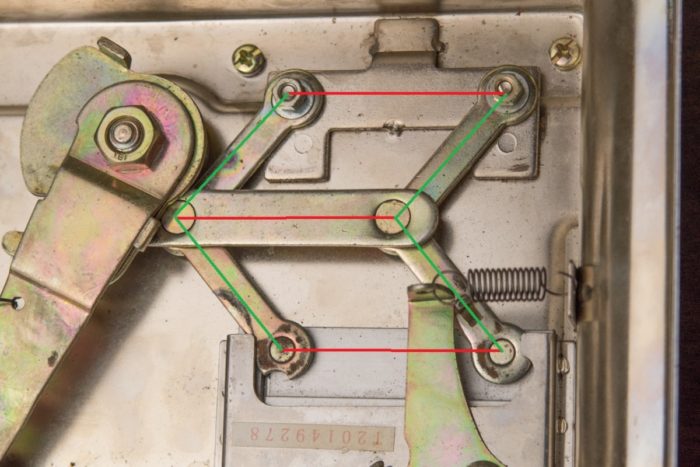
Parallel linkage is probably the most recognizable method of keeping level and the easiest to design. Two equal length parallel links are hinged to fixed pivot points. The line drawn between the two pivot points is the line we will remain parallel with as we rotate the links. Please note that the links do not have to be straight. They are two force members (like a rope in tension) so if they are not straight (banana shaped), you will have additional moments to calculate. It is also self-evident that you cannot get continuous rotation with this system because the links will interfere with each other.
There are two main things to consider about designing a system of parallel links. They are load magnitude and load placement.
The placement of the load has no effect on how hard it is (for the cylinder) to lift up the load. It seems backwards, but the cylinder is only responsible for lifting the magnitude of the load. The placement of the load is managed by altering the force in the links. This is why you often see small cylinders lifting large loads. Let’s dive deeper into the math.
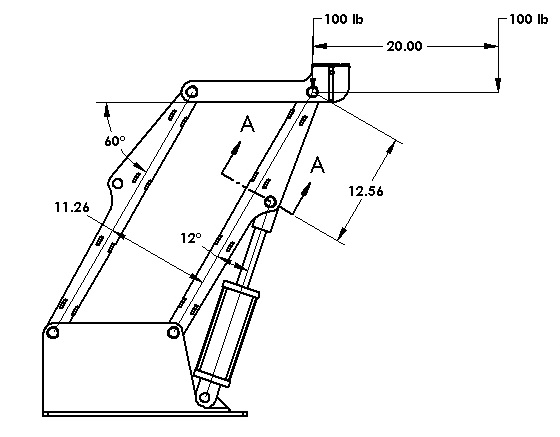
So, I’m going to prove to you that the placement of the load does effect something, but it doesn’t affect our cylinder load. For my first case, I’m going to put one hundred pounds right over the pin, and calculate the tension in the member left link. Doing the statics you need to sum the forces at the pin where the load is applied. The moment is the load (100 lb) times its moment arm (0 in) divided by the distance between the bars (11.26 in). Anything multiplied by zero is still zero, so there’s no force in that link. It’s kind of along for the ride and it’s just making sure that the bar maintains level.
I will now move my load out 20 inches and recalculate. I’ve now got a moment of 100 pounds times 20 inches or 2,000 in-lb. If I divide that by 11.26 in (distance between the links), my force will be 178 lb. So, I’ve increased the link load significantly by moving it out 20 inches. The tension member is very easy to calculate, but the compression member is a little more complicated because of the cylinder. The statics on the compression link is a little out of the subject so I won’t cover it in this article. It isn’t too difficult to calculate anyway.
Now let’s move onto the calculation of the cylinder force. It is largely a function of what the moment is at Section A-A. This moment is equal to the distance (12.56 in) times the load (100 lb) times the perpendicular angle between the two (60°) which equals 628 in-lb. Because of the pivot point at the end of the link, there will never be an increase if the load doesn’t increase.
To say it another way, the shear load in the top member is always 100 lb, but the moment load changes. We are only concerned with the shear load in this calculation. (Please note that the moment at A-A will change as the angle changes, so be careful). With linkage, you can get away with a relatively small cylinder to lift heavy loads because of this principle.
Warning: Load Placement is Critical
You can actually double the loads in a link if you are not careful. In the example above, I may have a load that centers on the pin and through its travels, the load may actually slide to the left or right. If we were using this to load baggage onto an airplane, we would have loads that shifted from the center to the edge of the structure. I mention this because we always want to have the cylinder interface with the compression link and we always want the compression link to stay in compression.
The reason that it matters is simple statics. Looking at Figure 2, the stress flow is clear. Our cylinder is in compression (if I remove it, the structure will fall to the right). As long as the link is in compression, the stress will flow nicely as shown with the thick line. However, not all of this load will flow through the cylinder (especially as the angle changes) and a small amount will be directed to the link. The point is that we will have a smooth stress flow throughout the link and cylinder.

If I move the load further left of the left link, I will change the direction of force in the right link. The link with the cylinder is now in tension. (Note that if the load is between the links, both will be in compression). Now the cylinder is still in compression as it tries to hold the load up.
If we look at the modified stress flow in Figure 3, we see that the primary stress flow is between the two pivoting pins and it is in tension. However, the compressive load of the cylinder needs somewhere to go. It wants to go up to the top pivot, but there are no forces to balance with. It then needs to make a U-turn (drastic changes in stress direction are quite awful) and flow back to the lower pivot. This stress from the lower pivot to the cylinder mount is a tensile stress and will add to the tensile stress of the link.
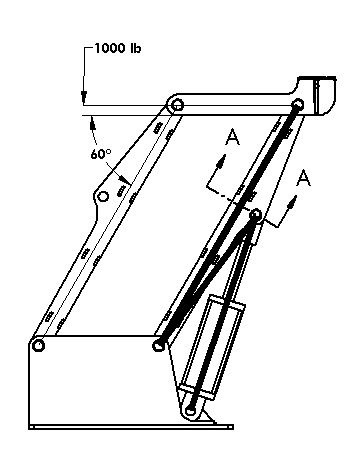
The result of the link being in tension is an amplification in stress. Also note that stresses don’t like making U-turns. By definition, it is a large stress concentration and will have you pulling out your hair in FEA (yup, been there). As a rule of thumb, always try to have your loads biased so that the member of the cylinder is in compression so that your links can be small and effective. In the rare case where I couldn’t prevent the reversing of loads, I was usually able to minimize the effects by limiting the offset distance and the magnitude of the load.
Conclusion
Parallel links are powerful tools and are used in many different types of machines. The benefits of using small cylinders to lift loads are self-evident and allow the lifting of heavy objects at longer distances.
Lifting objects is an essential part of engineering. Keeping them level throughout the lift is a welcomed challenge but can be stressful. After reading this article you should be able to take initial steps to designing a parallel linkage system.

